1.4 Activity: Solving Algebraic Equations-Making Connections to Balance Act
This activity will help us further our understanding of the role/meaning of equality sign by utilizing the balance scale with expressions to solve an algebraic equation and revisit the meaning of “solution of an equation.”
Part A
1) Go to the following link: Pan Balance – Expressions (Open in new window)
Follow the instructions to get familiar with the app, then complete the exploration. Provide your answers to the exploration questions #2-6 below :
2)
3)
4)
5)
6)
Try another equation and solve it using this app. Write your equation and solution below and explain how you used the app to solve it .
Part B
Review the following model (from Mathematics for Elementary Teachers by Sybilla Beckman), showing how to solve equations algebraically and using a pan balance. Consider the following questions:
(1) What did you learn about solving an equation?
(2) What similarities and differences do you see with this activity and the previous scale activities?
(3) How might an elementary school teacher use the Pan Balance to explain the process of solving an equation algebraically?
With a Pan Balance and Equations

[latex]4x+2+x=5+3x+3[/latex]
Change to equivalent expressions.

[latex]\begin{align*} 5x+2 &= 3x+8 \\ -2 &= -2 \\ \end{align*}[/latex]
Take 2 away from both sides.
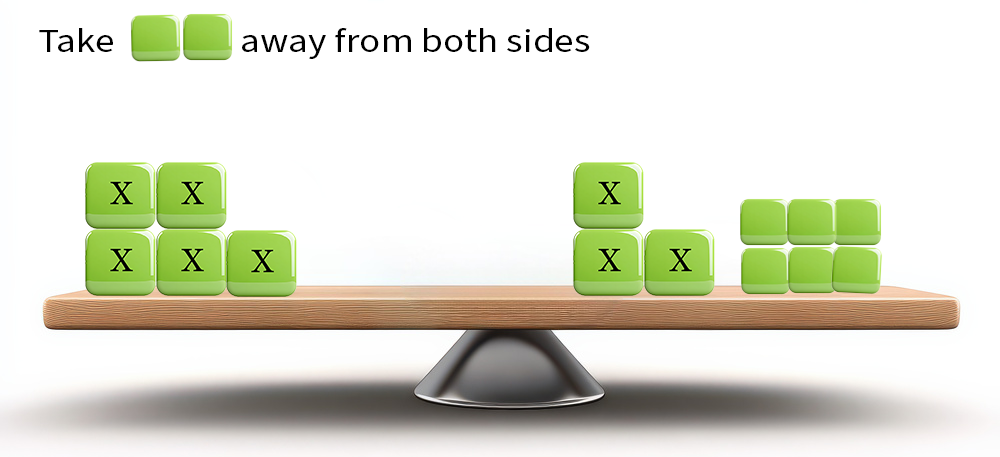
[latex]\begin{align*} 5x &= 3x+6 \\ -3x &= -3x \\ \end{align*}[/latex]
Take 3x away from both sides.

[latex]2x=6[/latex]
Divide both sides by 2.

[latex]x=3[/latex]
For extra explanation and examples watch the following videos:
Part C
Solve [latex]5x + 1 = 2x + 7[/latex] in two ways, (1) with equations and (2) with pictures of a pan balance. Relate the two methods with common explanations as done in Part B.
With equations:
[latex]5x + 1 = 2x + 7[/latex]
With a pan balance:




Part D
Write an equation involving variable x. Solve your equation in two ways: (1) with equations and (2) with the pictures of a pan balance. Relate the two methods with common explanations as done in Part B.
Chapter 1: Extra Exercises with more advanced equations, but first review corresponding algebraic properties as needed. (click on the triangle/arrow to expand)
1. Maria was given the following problem to solve: [latex]\frac{4x}{x+2}=3-\frac{8}{x+2}[/latex]
Her work is below. Explain what she did for each step and why her final answer is not valid.
[latex]4x = 3(x + 2) - 8[/latex]
[latex]4x = 3x + 6 - 8[/latex]
[latex]4x = 3x - 2[/latex]
The solution is [latex]x = -2[/latex]
2. Yihang was given the following problem to solve: [latex](\frac{1}{3}x-2)^{2}=\sqrt{4-x}[/latex]
His work is below. Explain what he did for each step and why his final answer is not valid.
[latex](\frac{1}{3}x-2)^{2}=4-x[/latex]
[latex]\frac{1}{9}x^{2}-\frac{4}{3}x+4=4-x[/latex]
[latex]x^2-12x+36=36-9x[/latex]
[latex]x^2-3x=0[/latex]
[latex]x(x-3)=0[/latex]
The solutions are [latex]x = 0[/latex] and [latex]x = 3[/latex].
3. For parts (a) – (c) an equation is given along with three attempts by various students to write an equivalent equation. For each student in each part, determine if their equation is equivalent to the original equation or not. Explain why or why not.
a) Original equation: [latex](x+3)^2=4x^2-36[/latex]
Alice: [latex]x+3=2x-6[/latex]
Bubba: [latex]x^2+9=4x^2-36[/latex]
Dalia: [latex]x^2=4x^2-39[/latex]
b) Original equation: [latex]x+\sqrt{x^2+9}=4[/latex]
Bubba: [latex]x^2+(x^2+9)=16[/latex]
Dalia: [latex]x+(x+3)=4[/latex]
c) Original equation: [latex]\frac{3x}{x+4}=2x-5[/latex]
Bubba: [latex]\frac{3}{4}=2x-5[/latex]
Dalia: [latex]3x=2x-5(x+4)[/latex]
a statement (with an equal sign) asserting that two expressions have the same numerical value
equal in value or meaning

